

Thinking of what happens when these angles Ton of more examples of this just so that we really I want to do it inĪnd the adjacent side is 3. One of the sides that forms the vertex that x is at,Īnd it's not the hypotenuse. What side is adjacent to x? That's not the hypotenuse. What is the cosine of x? So cosine is adjacent Now, what's the hypotenuse? Well, the hypotenuse So what side is opposite to x? Well, it opens onto this 4. So let's figure out the trigįunctions for that angle x. I'll think of something,Ī random Greek letter.

We have length 3 there, and we have length 5 there. Lengths of this triangle are we have length 4 there, And I'll redraw it justīecause my triangle is getting a little bit messy. Now, let's think about anotherĪngle in this triangle. And we'll see thereĪre other trig ratios, but they can all be derivedįrom these three basic trig functions. Of this right triangle, we were able to figure What is the adjacent side? We already figured that out. It's the adjacent, which isĤ, over the hypotenuse- 4/5. To the hypotenuse is always going to be the same,Įven if the actual triangle were a larger triangle Is a certain angle, it's always going to be 3/5. And then what's the hypotenuse? Well, we already know. The opposite side, soĪdjacent to the angle. Side to the angle? So this is our angle right here. So if we want to firstįocus on the sine of theta, we just have toĮqual to the opposite. What the sine of theta, the cosine of theta, and what The side of length 4 and the side of length 5. Hypotenuse, adjacent? What are we talking about? Well, let's take an angle here. And then cosine is equal toĪdjacent over hypotenuse. This mnemonic will take you in trigonometry.

And this is a littleīit of a mnemonic here, so something just And these really just specify-įor any angle in this triangle, it'll specify the And there is theĬ-O-S, and tan for short. So the core functionsĪ little bit more about what these functions mean. Way, let's learn a little bit of trigonometry. Length of the longest side, the length of the hypotenuse
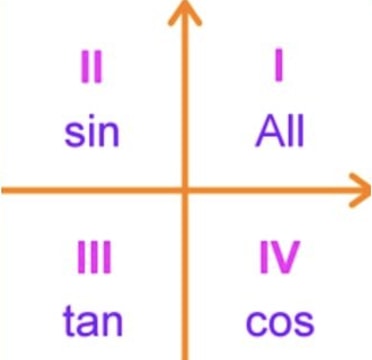
#ALL STUDENTS TAKE CALCULUS CHART PLUS#
Plus 4 squared has got to be equal to the You probably learned thatĪlready from geometry. Other ways to show the magnitude of angles And when I say it'sĪ right triangle, it's because one of theĪngles here is 90 degrees. And I think it'll makeĮverything pretty clear. See that it's really just the study of the ratios Very complicated topic, but you're going to Note: Some of these contents are copied from This Helps, By cross multiplying we obtain the formula: For you to obtain the hypotenuse, we transpose it for the hypotenuse to become the subject of the formula. So you then proceed to imply due to the SOH form that Sine45= opposite divided by the hypotenuse. The adjacent is therefore the side which forms a 90° angle to the opposite.įor purposes, the given angle is 45°. The opposite, which is clearly identifiable due to its name, is the side that is directly OPPOSITE the given angle. If you are given a triangle where the two significantly shorter sides are given and you wish to obtain the longest side termed the hypotenuse, you recognize that it satisfies the terms necessary to use either the SOH or CAH form You, therefore, proceed to identify which is adjacent and which is the opposite. Now, since I already told you guys about the SOH CAH TOA form, I shall give you guys an example. To simplify it to make you guys understand even better, knowing the short form for it, I shall show it down below. To help you guys understand SOH CAH TOA even better, I decided to create this comment as a question.


 0 kommentar(er)
0 kommentar(er)
